

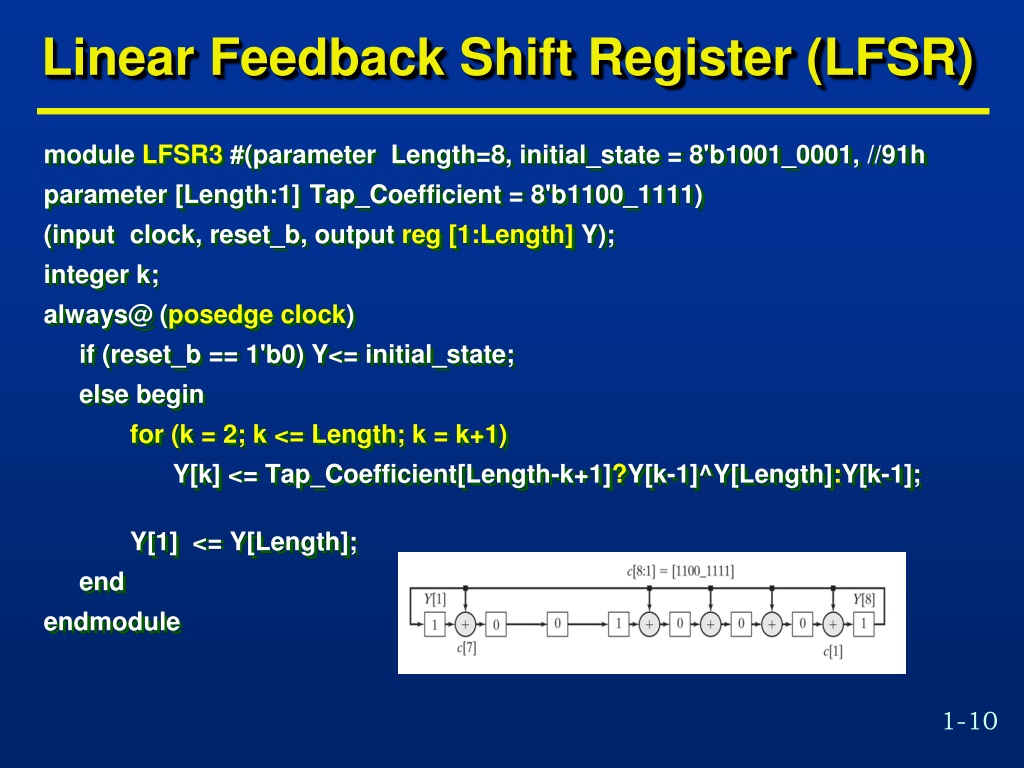
explained for the per iods, cycle sets and sequence struc tures, where the characteristic. Here's some example code that will do the job. The non-linear feedback shift registers used for the security techniques (cryptographic. D flip-flop is playing a major task in the LFSRs. Power Optimization Techniques: Linear feedback shift register is the main component used in self-tests of integrated circuits. To obfuscate a series of byte values, you might find it easier to extract just 8 bits at each iteration. proposed methodology is explained in section III and the results are analyzed in the section IV. Just XOR these values with your data to obfuscate it, and repeat the process using the same sequence of numbers to retrieve the original data. ICEPOLE works on the 1280-bit state and the reason for that is explained above in. A widely used example of the latter is the Mersenne twister, MT19937, invented by Matsumoto and Nishimura. The polynomial representation of LFSR is 圆4 + 圆3 + 圆1 + 圆0 + 1.
Obfuscating data with an LFSR:Īs long as your LSFR initially contains a non-zero value, it will step through a sequence of 65535 (2 16–1) pseudorandom values at every iteration. There are more complicated linear generators, e.g., matrix multiplicative recursive generators, generalized feedback shift registers, and twisted generalized feedback shift registers, but we do not discuss them. The list of 16-bit LFSRs has 2048 entries. This page contains lists of feedback constants that work with LFSRs of different lengths. (Note: There is no XOR gate feeding into bit 16 because there is no input from bit 17.) Other LFSRs: The quickest way of doing this is by calculating the XOR product of the 16-bit shift register with 0xB400, which has all of these bits set (1011010000000000 in binary). When this bit is zero, this has no effect, but when it is 1, all these bits are flipped. The least significant bit extracted from the end of the shift register is fed back by XOR-ing it with bits 16, 14, 13 and 11. One of the more common forms of LFSR is formed from a simple shift register with feedback from two or more points, or taps, in the register chain (Fig 1). That one is handy for software implementations, for the representation of the polynomials can be compressed into a small bitmask.The Wikipedia article you got this from has an illustration that explains what the code is doing: LFSRs are simple to construct and are useful for a wide variety of applications, but are often sadly neglected by designers. Reference is given to: Janusz Rajski et Jerzy Tyszer, Primitive Polynomials Over GF(2) of Degree up to 660 with Uniformly Distributed Coefficients, which free main table there (part 1, 2 3).įurther, highbit-normalprimpoly gives one primitive polynomial per degree up to 400, selected has having the lowest non-constant term as high as possible.
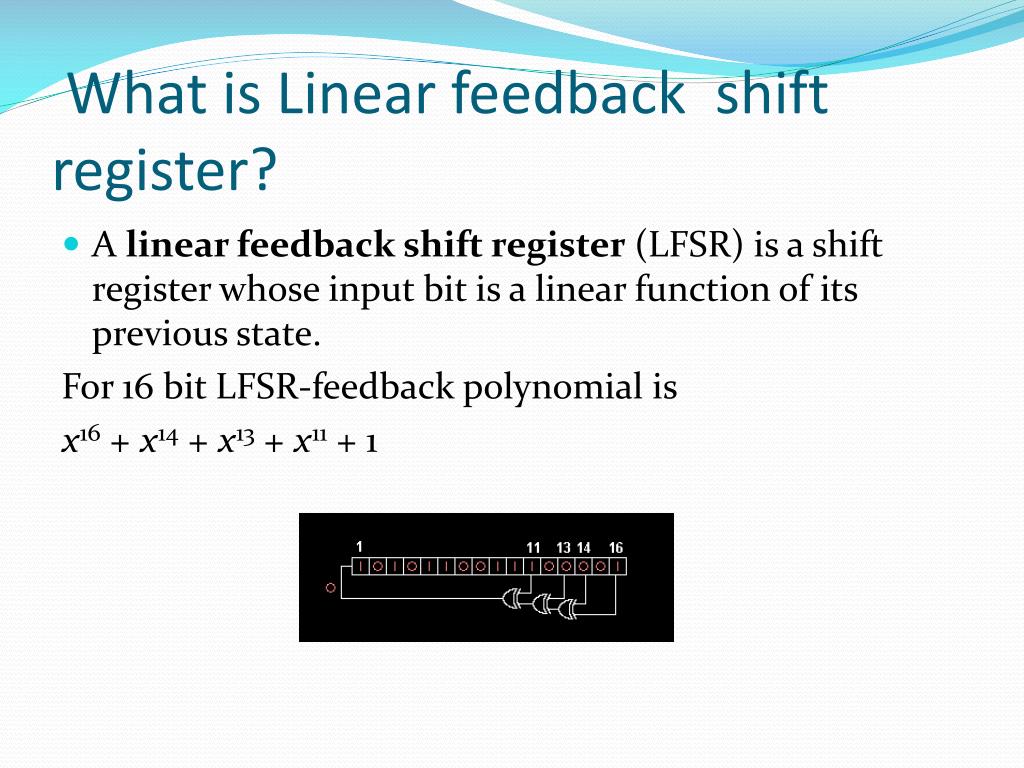
In particular, all-trinomial-primpoly gives primitive GF(2) trinomials (LFSR with 2 taps, the minimum number) to degree 400. When I need something on that tune I might use Jörg's useful and ugly page of mathematical data.


 0 kommentar(er)
0 kommentar(er)
